Difference between revisions of "Cheat sheet/ko"

Revision as of 13:26, 10 October 2014 (view source) Ysjbserver (talk | contribs) m ← Older edit		Latest revision as of 11:09, 15 May 2021 (view source) Pogo (talk | contribs) (Added category.)
(One intermediate revision by one other user not shown)
Line 7:		Line 7:
	===추력비(Thrust to Weight Ratio; TWR)===		===추력비(Thrust to Weight Ratio; TWR)===
	{{See also|Thrust-to-weight ratio}}		{{See also|Thrust-to-weight ratio}}
−	This is Newton's Second Law. If the ratio is less than 1 the craft will not lift off the ground. Note that the local gravitational acceleration, which is usually the surface gravity of the body the rocket is starting from, is required.	+	이건 제 2 뉴턴 운동 법칙를 따릅니다. 만약 이 값이 1보다 작다면, 땅을 떠나지 못하겠죠. 발사하는 곳의 표면 기준의 중력가속도 값이 필요하다는 것을 잘 알아두세요!
−	{{Formula|math=\text{TWR} = \frac{F_T}{m \cdot g} > 1|where=* <math>F_T</math> is the thrust of the engines	+	{{Formula|math=\text{TWR} = \frac{F_T}{m \cdot g} > 1|where=* <math>F_T</math> 엔진의 출력
−	* <math>m</math> the total mass of the craft	+	* <math>m</math> 전체 중량
−	* <math>g</math> the local gravitational acceleration (usually surface gravity)}}	+	* <math>g</math> 중력 가속도 값}}
	===합산비추력(Combined Specific Impulse; I<sub>sp</sub>)===		===합산비추력(Combined Specific Impulse; I<sub>sp</sub>)===
Line 165:		Line 165:
	* [[Terminology]]		* [[Terminology]]
	* [[thread:28352|The Drawing Board: A library of tutorials and other useful information]]		* [[thread:28352|The Drawing Board: A library of tutorials and other useful information]]
		+
		+	[[Category: Articles/ko]]

---

Latest revision as of 11:09, 15 May 2021

경고: 평서체로 번역할 경우, 바로 삭제 신청이 들어갈 것입니다. Ysjbserver (talk)

Kerbal Space Program rocket scientist's cheat sheet: Delta-v maps, equations and more for your reference so you can get from here to there and back again.

수학

추력비(Thrust to Weight Ratio; TWR)

→ 참고하기: Thrust-to-weight ratio

이건 제 2 뉴턴 운동 법칙를 따릅니다. 만약 이 값이 1보다 작다면, 땅을 떠나지 못하겠죠. 발사하는 곳의 표면 기준의 중력가속도 값이 필요하다는 것을 잘 알아두세요!

합산비추력(Combined Specific Impulse; I_sp)

→ 참고하기: Specific impulse

If the I_sp is the same for all engines in a stage, then the I_sp is equal to a single engine. If the I_sp is different for engines in a single stage, then use the following equation:

${\displaystyle I_{sp}={\frac {(F_{1}+F_{2}+\dots )}{{\frac {F_{1}}{I_{sp1}}}+{\frac {F_{2}}{I_{sp2}}}+\dots }}}$

델타V (Delta-v; Δv)

Basic calculation

→ 참고하기: Tutorial:Advanced Rocket Design

Basic calculation of a rocket's Δv. Use the atmospheric and vacuum thrust values for atmospheric and vacuum Δv, respectively.

True Δv of a stage that crosses from atmosphere to vacuum

Body	Δvout
Kerbin	1000 m/s
other bodies' data missing

Calculation of a rocket stage's Δv, taking into account transitioning from atmosphere to vacuum. Δv_out is the amount of Δv required to leave a body's atmosphere, not reach orbit. This equation is useful to figure out the actual Δv of a stage that transitions from atmosphere to vacuum.

${\displaystyle \Delta {v}_{T}={\frac {\Delta {v}_{atm}-\Delta {v}_{out}}{\Delta {v}_{atm}}}\cdot \Delta {v}_{vac}+\Delta {v}_{out}}$

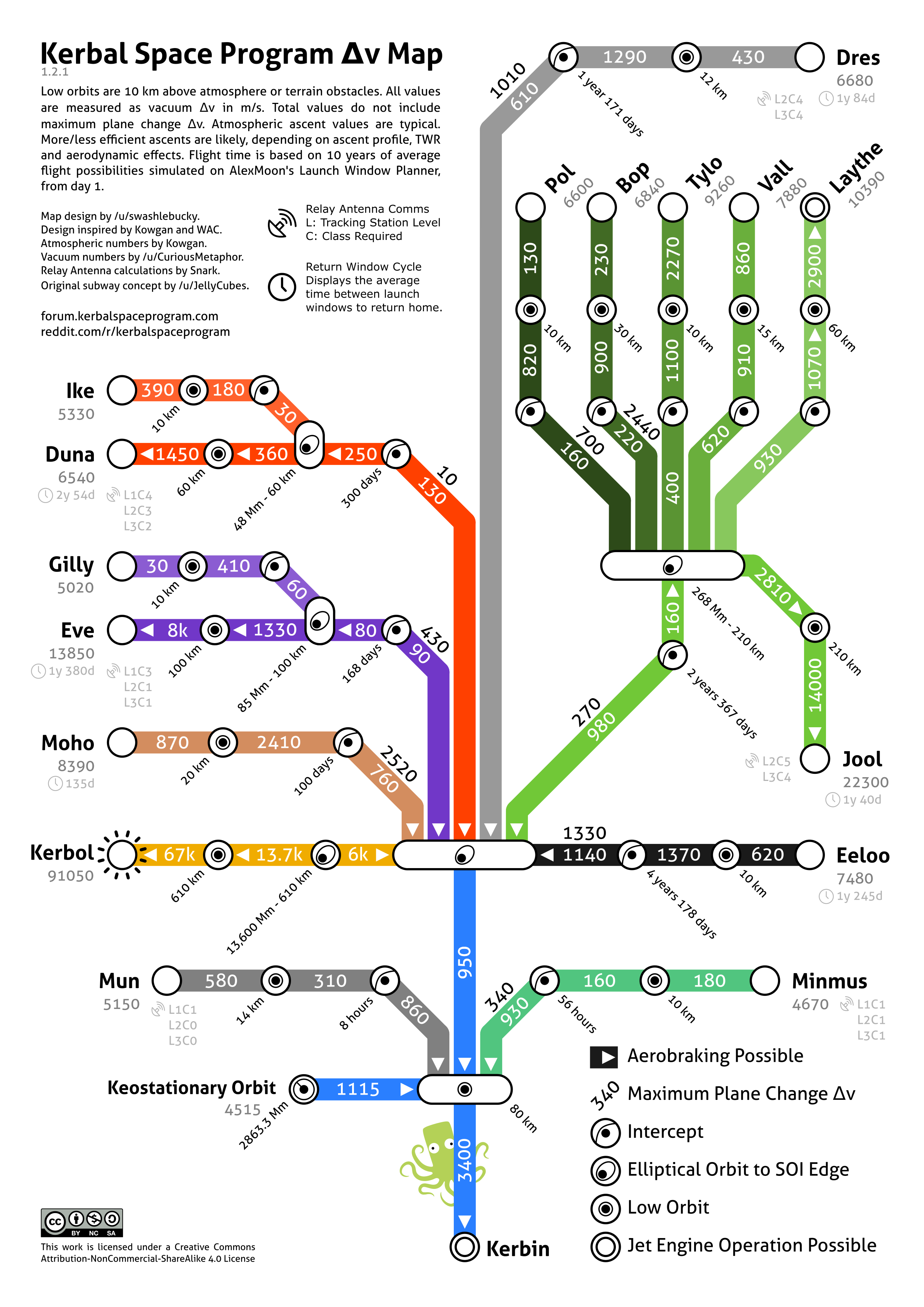
Maps

Various fan-made maps showing the Δv required to travel to a certain body.

Subway style Δv map:

Total Δv values

• http://www.skyrender.net/lp/ksp/system_map.png

Δv change values

• http://i.imgur.com/duY2S.png

Δv nomogram

• http://ubuntuone.com/1kD39BCoV38WP1QeG6MtO6

Δv with Phase Angles

• http://i.imgur.com/dXT6r7s.png

Precise Total Δv values

• http://i.imgur.com/UUU8yCk.png

Maximum Δv Chart

This chart is a quick guide to what engine to use for a single stage interplanetary ship. No matter how much fuel you add you will never reach these ΔV without staging to shed mass or using the slingshot maneuver.

ISP (s)	Max Δv (m/s)	Engines
290	6257	LV-1 LV-1R
300	6473	24-77
320	6905	Mark-55
330	7120	Mainsail
350	7552	Skipper 48-7S
360	7768	KS-25X4 KR-1X2
370	7983	LV-T30 LV-T45
380	8199	KR-2L
390	8415	Poodle LV-909 Aerospike
800	17261	LV-N

Math examples

TWR

• Copy template:

TWR = F / (m * g) > 1

I_sp

1. When I_sp is the same for all engines in a stage, then the I_sp is equal to a single engine. So six 200 I_sp engines still yields only 200 I_sp.
2. When I_sp is different for engines in a single stage, then use the following equation:

• Equation:

${\displaystyle I_{sp}={\frac {(F_{1}+F_{2}+\dots )}{{\frac {F_{1}}{I_{sp1}}}+{\frac {F_{2}}{I_{sp2}}}+\dots }}}$

• Simplified:

I_sp = ( F1 + F2 + ... ) / ( ( F1 / I_sp1 ) + ( F2 / I_sp2 ) + ... )

• Explained:

I_sp = ( Force of Thrust of 1st Engine + Force of Thrust of 2nd Engine...and so on... ) / ( ( Force of Thrust of 1st Engine / I_sp of 1st Engine ) + ( Force of Thrust of 2nd Engine / I_sp of 2nd Engine ) + ...and so on... )

• Example:

Two engines, one rated 200 newtons and 120 seconds I_sp ; another engine rated 50 newtons and 200 seconds I_sp.

Isp = (200 newtons + 50 newtons) / ( ( 200 newtons / 120 ) + ( 50 newtons / 200 ) = 130.89 seconds I_sp

Δv

1. For atmospheric Δv value, use atmospheric thrust values.
2. For vacuum Δv value, use vacuum thrust values.
3. Use this equation to figure out the Δv per stage:

• Equation:

${\displaystyle \Delta {v}=ln\left({\frac {M_{start}}{M_{dry}}}\right)\cdot I_{sp}\cdot 9.81{\frac {m}{s^{2}}}}$

• Simplified:

Δv = ln ( Mstart / Mdry ) * I_sp * g

• Explained:

Δv = ln ( Starting Mass / Dry Mass ) X Isp X 9.81

• Example:

Single Stage Rocket that weighs 23 tons when full, 15 tons when fuel is emptied, and engine that outputs 120 seconds I_sp.

Δv = ln ( 23 Tons / 15 Tons ) × 120 seconds I_sp × 9.81m/s² = Total Δv of 503.2 m/s

Maximum Δv

Simplified version of the Δv calculation to find the maximum Δv a craft with the given ISP could hope to achieve. This is done by using a magic 0 mass engine and not having a payload.

• Equation:

${\displaystyle \Delta {v}=21.576745349086\cdot I_{sp}}$

• Simplified:

Δv =21.576745349086 * I_sp

• Explained / Examples:

This calculation only uses the mass of the fuel tanks and so the ln ( Mstart / Mdry ) part of the Δv equation has been replaced by a constant as Mstart / Mdry is always 9 (or worse with some fuel tanks) regardless of how many fuel tanks you use.

The following example will use a single stage and fuel tanks in the T-100 to Jumbo 64 range with an engine that outputs 380 seconds I_sp.

Δv = ln ( 18 Tons / 2 Tons ) × 380 seconds I_sp × 9.81m/s² = Maximum Δv of 8199.1632327878 m/s

Δv = 2.1972245773 × 380 seconds I_sp × 9.82m/s² = Maximum Δv of 8199.1632327878 m/s (Replaced the log of mass with a constant as the ratio of total mass to dry mass is constant regardless of the number of tanks used as there is no other mass involved)

Δv = 21.576745349086 × 380 seconds I_sp = Maximum Δv of 8199.1632327878 m/s (Reduced to its most simple form by combining all the constants)

True Δv

1. How to calculate the Δv of a rocket stage that transitions from Kerbin atmosphere to vacuum.
2. Assumption: It takes approximately 1000 m/s of Δv to escape Kerbin's atmosphere before vacuum Δv values take over for the stage powering the transition.
3. Note: This equation is an guess, approximation, and is not 100% accurate. Per forum user stupid_chris who came up with the equation: "The results will vary a bit depending on your TWR and such, but it should usually be pretty darn accurate."

• Equation for Kerbin Atmospheric Escape:

${\displaystyle \Delta {v}_{T}={\frac {\Delta {v}_{atm}-\Delta {v}_{out}}{\Delta {v}_{atm}}}\cdot \Delta {v}_{vac}+\Delta {v}_{out}}$

• Simplified:

True Δv = ( ( Δv atm - 1000 ) / Δv atm ) * Δv vac + 1000

• Explained:

True Δv = ( ( Total Δv in atmosphere - 1000 m/s) / Total Δv in atmosphere ) X Total Δv in vacuum + 1000

• Example:

Single Stage with total atmospheric Δv of 5000 m/s, and rated 6000 Δv in vacuum.

Transitional Δv = ( ( 5000 Δv atm - 1000 Δv Required to escape Kerbin atmosphere ) / 5000 Δv atm ) X 6000 Δv vac + 1000 Δv Required to escape Kerbin atmosphere = Total Δv of 5800 m/s

See also

• Tutorials
• Terminology
• The Drawing Board: A library of tutorials and other useful information