Difference between revisions of "Tutorial: Basic Orbiting (Technical)"
(→Formulae and Tables) |
SlayerGames (talk | contribs) m (Fixed categories) |
||
| Line 1: | Line 1: | ||
| − | + | {{Stub|tutorial|Needs some math tags and general cleanup. -- [[User:N3X15|N3X15]] ([[User talk:N3X15|talk]]) 08:46, 1 October 2012 (UTC)}} | |
| − | |||
| − | + | Getting into space is relatively easy, but staying there without drifting endlessly into space or falling back down to Kerbin can be challenging. This tutorial will teach you how to get into and remain in [[orbit]], how to adjust your orbit to be circular or elliptical, and how to adjust to a higher or lower orbit. | |
| − | |||
| − | + | == Stabilizing your orbit == | |
| − | |||
| − | |||
| − | + | During each orbit, your craft will reach maximum altitude, called '''apoapsis''', and on the opposite side of the planet, it will reach minimum altitude, called '''periapsis'''. At both apoapsis and periapsis, your vertical speed will be zero. These points are the easiest points to make orbital corrections, because you can easily determine how fast to go when your vertical speed is zero. '''Note:''' The relative difference between your orbit's apoapsis and periapsis is called its '''eccentricity.''' Orbits that are exactly circular have zero eccentricity, and highly "flattened-out" orbits have eccentricity close to 1. | |
| − | + | There are a number of third-party calculators available which can crunch the numbers and tell you your eccentricity, as well as provide the speeds required to circularize your orbit at your current (or future) altitude. Whether you calculate your orbits by hand, or use a third party app, the general procedures are still the same and are given below: | |
| − | + | First, in order to get into a nice, round orbit, you need to determine how fast to go. The mathematical basis for orbital speed is determined from your current distance from your central body (<math>r</math>), your [[semi-major axis]] from your central body (<math>a</math>), and the mass of the central body itself (<math>M</math>). These may be use to find the speed at an orbit around any body using the relation | |
| − | |||
| − | + | <math>v = \sqrt{GM\left(\frac{2}{r}-\frac{1}{a}\right)}</math> | |
| − | + | where <math>G</math> is the [[w:gravitational constant|gravitational constant]] <math>6.674 \cdot 10^{-11}\mathrm{\frac{m^3}{kg \cdot s^2}}</math>. Keep in mind that distances to the central body must account not only for altitude but also for the radius (<math>R</math>) of whatever body you are orbiting. The exact values of <math>M</math> and <math>R</math> may be found on their respective pages. | |
| − | [ | ||
| − | + | Returning to our case, the higher your orbit, the less gravity you'll feel from Kerbin, so the slower you'll need to go to be in a circular orbit. Determine the proper speed for your altitude at apoapsis or periapsis either by hand, by calculator, or by table. You'll probably want to watch your altimeter as you near one of the critical points, remember the altitude, determine your desired speed, and make the correction on your next pass. If you want to "round out" your orbit from apoapsis, you need to speed up to avoid falling back down to periapsis. Point your craft in the exact direction of travel (use the chartreuse yellow circular indicator on the [[Navball]] to line up), and apply thrust until you've gained enough speed. To round out an orbit from periapsis, you need to slow down to avoid climbing back up to apoapsis. Point your craft in the opposite direction of travel (indicated on the Navball by a chartreuse yellow circle with an "X" through it), and apply thrust until you have slowed to the speed indicated by the table. You should then be in an orbit that is very close to circular! Depending on how eccentric your initial orbit was, you may need to make a large correction on your first pass followed by a small correction on a subsequent pass to get very stable. | |
| − | |||
| − | + | For fine adjustments to your orbit, adding a set of [[RCS]] thrusters to your craft helps immensely. Additionally, you can see the current trajectory (and read periapsis and apoapsis altitudes) by switching to the [[Map view]] (M key). | |
| − | + | == Transfer Orbits == | |
| − | + | The most efficient way to transfer from a lower circular orbit to a higher circular orbit (or vice-versa) is to use an elliptical transfer orbit, also known as a Hohmann transfer orbit. To transfer, we make the periapsis of the elliptical orbit the same as the radius of the lower orbit, and the apoapsis of the elliptical orbit the same as the radius of the higher orbit. If you are going from low to high, you make a burn in the direction of travel to elongate your orbit. You will climb in altitude as you travel around the planet to the apoapsis of your transfer orbit. Then, make a second burn to round out the new, higher orbit (as described above). To go from high to low, do the opposite: Burn in the opposite direction of travel, then fall down to the periapsis of your transfer orbit, and make a second burn to round out the lower orbit (again in the opposite direction of travel). | |
| − | + | === Target Speed === | |
| − | + | The key to transfer orbits is figuring out how much speed to add or subtract to reach a desired new orbital altitude. To do this, use the formula below to determine the target velocity for your initial burn: | |
| − | + | <math>v = 1,878,968 \cdot \sqrt{\frac{2}{r_i} - \frac{2}{(r_l+r_h)}}</math> | |
| − | + | In this formula, ''r<sub>l</sub>'' and ''r<sub>h</sub>'' are the radii of the lower and higher orbits, respectively, and ''r<sub>i</sub>'' is the radius of the initial orbit. If you are transferring to a higher orbit, ''r<sub>i</sub>'' will be equal to ''r<sub>l</sub>'', and ''v'' will be faster than your current speed, so burn in the direction of travel to reach ''v''. If you are transferring to a lower orbit, ''r<sub>i</sub>'' will be equal to ''r<sub>h</sub>'', and ''v'' will be slower than your current speed, so burn in the opposite direction to reach ''v''. Remember, ''v'' is the target speed for your initial burn that puts you into the elliptical transfer orbit. Once you reach your new orbital altitude, you need to make a second burn to round out your orbit, using the same technique described in the [[Tutorial: Basic Orbiting#Stabilizing your orbit|stabilizing your orbit]] section. | |
| − | ' | + | Details of where this formula comes from are in the technical section below. When using this formula, take care to remember that the radius of an orbit is equal to the orbital altitude plus Kerbin's radius (600 000 m). |
| − | + | === De-orbiting === | |
| + | The most efficient way to de-orbit from any altitude is to initiate a transfer orbit with a periapsis below 69076 m, the edge of Kerbin's atmosphere. Note that the upper atmosphere is very thin so if you do not want to wait for several orbits of [[aerobraking]], aim for under 35000 m and thicker air. As you approach periapsis, the atmospheric drag will start to slow your craft and eventually it can no longer maintain orbit. | ||
| − | + | === R code snippet for planning Hohmann transfer === | |
| − | + | hohmann <- function(from_alt,to_alt){ | |
| + | # provides information needed to perform | ||
| + | # a hohmann transfer from a circular ortbit | ||
| + | # at from_alt (km) to a circular orbit at to_alt (km) | ||
| + | mu <- 3531.6 # Gravitational parameter (km^3/s^2) | ||
| + | R <- 600 # Kerbin radius (km) | ||
| + | r1 <- from_alt+R # radius 1 (km) | ||
| + | r2 <- to_alt+R # radius 2 (km) | ||
| + | vc1 <- sqrt(mu/r1) # circular orbit velocity 1 (km/s) | ||
| + | vc2 <- sqrt(mu/r2) # circular orbit velocity 2 (km/s) | ||
| + | a <- (r1+r2)/2 # semi-major axis of transfer orbit (km) | ||
| + | T <- 2*pi*sqrt((a^3)/mu) # period of transfer orbit (s) | ||
| + | dv1 <- (sqrt(r2/a)-1)*vc1 # delta v1 (km/s) | ||
| + | dv2 <- (1-sqrt(r1/a))*vc2 # delta v2 (km/s) | ||
| + | b1 <- list(from=vc1,to=vc1+dv1) # burn one from-to velocities (km/s) | ||
| + | t <- T/2 # time between burns (s) | ||
| + | b2 <- list(from=vc2+dv2,to=vc2) # burn two from-to velocities (km/s) | ||
| + | out <- list(from_alt=from_alt,b1=b1,t=t,b2=b2,to_alt=to_alt) | ||
| + | return(out)} | ||
| − | + | ==== Example usage ==== | |
| − | + | Produce a graph showing the speeds need to transfer from a range of circular orbit altitudes into a landing orbit. | |
| + | |||
| + | plot(100*1:40,1000*hohmann(100*1:40,34)$b1$to,main="Landing speeds",xlab="altitude (km)",ylab="speed (m/s)") | ||
| + | |||
| + | [[File:landingspeeds.png]] | ||
| + | |||
| + | R project Link [http://www.r-project.org/] | ||
| + | |||
| + | |||
| + | === Transfer Orbits === | ||
| + | Coming soon! | ||
=== Orbital Table === | === Orbital Table === | ||
| − | '''Note:''' | + | '''Note:''' The atmosphere once had a sharp cutoff at 34.5 km, but now extends to approximately 69 km. Below this altitude, your orbit will gradually decay. The decay becomes quite rapid below about 45 km. The orbital parameters below 69 km are provided for reference, but understand that you will not be able to maintain these orbits without regular corrections to counteract the atmospheric drag. |
| − | + | {{:Tutorial: Basic Orbiting (Technical)/table|Altitude|Orbital speed|Orbital period}} | |
| − | + | ||
| − | + | [[Category:Tutorials|Basic Orbiting (Technical)]] | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | | | ||
| − | | | ||
| − | |||
| − | |||
| − | |||
| − | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
Latest revision as of 19:52, 2 May 2019
Getting into space is relatively easy, but staying there without drifting endlessly into space or falling back down to Kerbin can be challenging. This tutorial will teach you how to get into and remain in orbit, how to adjust your orbit to be circular or elliptical, and how to adjust to a higher or lower orbit.
Contents
Stabilizing your orbit
During each orbit, your craft will reach maximum altitude, called apoapsis, and on the opposite side of the planet, it will reach minimum altitude, called periapsis. At both apoapsis and periapsis, your vertical speed will be zero. These points are the easiest points to make orbital corrections, because you can easily determine how fast to go when your vertical speed is zero. Note: The relative difference between your orbit's apoapsis and periapsis is called its eccentricity. Orbits that are exactly circular have zero eccentricity, and highly "flattened-out" orbits have eccentricity close to 1.
There are a number of third-party calculators available which can crunch the numbers and tell you your eccentricity, as well as provide the speeds required to circularize your orbit at your current (or future) altitude. Whether you calculate your orbits by hand, or use a third party app, the general procedures are still the same and are given below:
First, in order to get into a nice, round orbit, you need to determine how fast to go. The mathematical basis for orbital speed is determined from your current distance from your central body (), your semi-major axis from your central body (), and the mass of the central body itself (). These may be use to find the speed at an orbit around any body using the relation
where is the gravitational constant . Keep in mind that distances to the central body must account not only for altitude but also for the radius () of whatever body you are orbiting. The exact values of and may be found on their respective pages.
Returning to our case, the higher your orbit, the less gravity you'll feel from Kerbin, so the slower you'll need to go to be in a circular orbit. Determine the proper speed for your altitude at apoapsis or periapsis either by hand, by calculator, or by table. You'll probably want to watch your altimeter as you near one of the critical points, remember the altitude, determine your desired speed, and make the correction on your next pass. If you want to "round out" your orbit from apoapsis, you need to speed up to avoid falling back down to periapsis. Point your craft in the exact direction of travel (use the chartreuse yellow circular indicator on the Navball to line up), and apply thrust until you've gained enough speed. To round out an orbit from periapsis, you need to slow down to avoid climbing back up to apoapsis. Point your craft in the opposite direction of travel (indicated on the Navball by a chartreuse yellow circle with an "X" through it), and apply thrust until you have slowed to the speed indicated by the table. You should then be in an orbit that is very close to circular! Depending on how eccentric your initial orbit was, you may need to make a large correction on your first pass followed by a small correction on a subsequent pass to get very stable.
For fine adjustments to your orbit, adding a set of RCS thrusters to your craft helps immensely. Additionally, you can see the current trajectory (and read periapsis and apoapsis altitudes) by switching to the Map view (M key).
Transfer Orbits
The most efficient way to transfer from a lower circular orbit to a higher circular orbit (or vice-versa) is to use an elliptical transfer orbit, also known as a Hohmann transfer orbit. To transfer, we make the periapsis of the elliptical orbit the same as the radius of the lower orbit, and the apoapsis of the elliptical orbit the same as the radius of the higher orbit. If you are going from low to high, you make a burn in the direction of travel to elongate your orbit. You will climb in altitude as you travel around the planet to the apoapsis of your transfer orbit. Then, make a second burn to round out the new, higher orbit (as described above). To go from high to low, do the opposite: Burn in the opposite direction of travel, then fall down to the periapsis of your transfer orbit, and make a second burn to round out the lower orbit (again in the opposite direction of travel).
Target Speed
The key to transfer orbits is figuring out how much speed to add or subtract to reach a desired new orbital altitude. To do this, use the formula below to determine the target velocity for your initial burn:
In this formula, rl and rh are the radii of the lower and higher orbits, respectively, and ri is the radius of the initial orbit. If you are transferring to a higher orbit, ri will be equal to rl, and v will be faster than your current speed, so burn in the direction of travel to reach v. If you are transferring to a lower orbit, ri will be equal to rh, and v will be slower than your current speed, so burn in the opposite direction to reach v. Remember, v is the target speed for your initial burn that puts you into the elliptical transfer orbit. Once you reach your new orbital altitude, you need to make a second burn to round out your orbit, using the same technique described in the stabilizing your orbit section.
Details of where this formula comes from are in the technical section below. When using this formula, take care to remember that the radius of an orbit is equal to the orbital altitude plus Kerbin's radius (600 000 m).
De-orbiting
The most efficient way to de-orbit from any altitude is to initiate a transfer orbit with a periapsis below 69076 m, the edge of Kerbin's atmosphere. Note that the upper atmosphere is very thin so if you do not want to wait for several orbits of aerobraking, aim for under 35000 m and thicker air. As you approach periapsis, the atmospheric drag will start to slow your craft and eventually it can no longer maintain orbit.
R code snippet for planning Hohmann transfer
hohmann <- function(from_alt,to_alt){
# provides information needed to perform
# a hohmann transfer from a circular ortbit
# at from_alt (km) to a circular orbit at to_alt (km)
mu <- 3531.6 # Gravitational parameter (km^3/s^2)
R <- 600 # Kerbin radius (km)
r1 <- from_alt+R # radius 1 (km)
r2 <- to_alt+R # radius 2 (km)
vc1 <- sqrt(mu/r1) # circular orbit velocity 1 (km/s)
vc2 <- sqrt(mu/r2) # circular orbit velocity 2 (km/s)
a <- (r1+r2)/2 # semi-major axis of transfer orbit (km)
T <- 2*pi*sqrt((a^3)/mu) # period of transfer orbit (s)
dv1 <- (sqrt(r2/a)-1)*vc1 # delta v1 (km/s)
dv2 <- (1-sqrt(r1/a))*vc2 # delta v2 (km/s)
b1 <- list(from=vc1,to=vc1+dv1) # burn one from-to velocities (km/s)
t <- T/2 # time between burns (s)
b2 <- list(from=vc2+dv2,to=vc2) # burn two from-to velocities (km/s)
out <- list(from_alt=from_alt,b1=b1,t=t,b2=b2,to_alt=to_alt)
return(out)}
Example usage
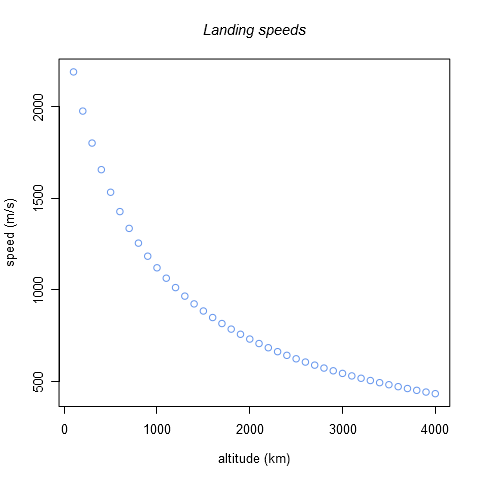
Produce a graph showing the speeds need to transfer from a range of circular orbit altitudes into a landing orbit.
plot(100*1:40,1000*hohmann(100*1:40,34)$b1$to,main="Landing speeds",xlab="altitude (km)",ylab="speed (m/s)")
R project Link [1]
Transfer Orbits
Coming soon!
Orbital Table
Note: The atmosphere once had a sharp cutoff at 34.5 km, but now extends to approximately 69 km. Below this altitude, your orbit will gradually decay. The decay becomes quite rapid below about 45 km. The orbital parameters below 69 km are provided for reference, but understand that you will not be able to maintain these orbits without regular corrections to counteract the atmospheric drag.
| Altitude (m) | Orbital speed (m/s) | Orbital period |
|---|---|---|
| 40 000 | 2 349.1 | 28 m 31.8 s |
| 50 000 | 2 330.9 | 29 m 12.1 s |
| 60 000 | 2 313.2 | 29 m 52.7 s |
| 70 000 | 2 295.9 | 30 m 33.6 s |
| 80 000 | 2 278.9 | 31 m 14.8 s |
| 90 000 | 2 262.4 | 31 m 56.3 s |
| 100 000 | 2 246.1 | 32 m 38.1 s |
| 110 000 | 2 230.3 | 33 m 20.2 s |
| 120 000 | 2 214.7 | 34 m 2.6 s |
| 130 000 | 2 199.5 | 34 m 45.3 s |
| 140 000 | 2 184.6 | 35 m 28.3 s |
| 150 000 | 2 170.0 | 36 m 11.6 s |
| 160 000 | 2 155.7 | 36 m 55.2 s |
| 170 000 | 2 141.6 | 37 m 39.1 s |
| 180 000 | 2 127.8 | 38 m 23.2 s |
| 190 000 | 2 114.3 | 39 m 7.7 s |
| 200 000 | 2 101.1 | 39 m 52.4 s |
| 210 000 | 2 088.1 | 40 m 37.4 s |
| 220 000 | 2 075.3 | 41 m 22.6 s |
| 230 000 | 2 062.8 | 42 m 8.2 s |
| 240 000 | 2 050.4 | 42 m 54 s |
| 250 000 | 2 038.3 | 43 m 40.1 s |
| 260 000 | 2 026.5 | 44 m 26.5 s |
| 270 000 | 2 014.8 | 45 m 13.1 s |
| 280 000 | 2 003.3 | 46 m 0.1 s |
| 290 000 | 1 992.0 | 46 m 47.2 s |
| 300 000 | 1 980.9 | 47 m 34.7 s |
| 310 000 | 1 970.0 | 48 m 22.4 s |
| 320 000 | 1 959.3 | 49 m 10.4 s |
| 330 000 | 1 948.7 | 49 m 58.6 s |
| 340 000 | 1 938.3 | 50 m 47.1 s |
| 350 000 | 1 928.1 | 51 m 35.8 s |
| 360 000 | 1 918.0 | 52 m 24.9 s |
| 370 000 | 1 908.1 | 53 m 14.1 s |
| 380 000 | 1 898.3 | 54 m 3.6 s |
| 390 000 | 1 888.7 | 54 m 53.4 s |
| 400 000 | 1 879.3 | 55 m 43.4 s |
| 410 000 | 1 869.9 | 56 m 33.7 s |
| 420 000 | 1 860.7 | 57 m 24.2 s |
| 430 000 | 1 851.7 | 58 m 15 s |
| 440 000 | 1 842.8 | 59 m 6 s |
| 450 000 | 1 834.0 | 59 m 57.3 s |
| 460 000 | 1 825.3 | 1 h 0 m 48.8 s |
| 470 000 | 1 816.7 | 1 h 1 m 40.6 s |
| 480 000 | 1 808.3 | 1 h 2 m 32.6 s |
| 490 000 | 1 800.0 | 1 h 3 m 24.8 s |
| 500 000 | 1 791.8 | 1 h 4 m 17.3 s |
| 510 000 | 1 783.7 | 1 h 5 m 10 s |
| 520 000 | 1 775.7 | 1 h 6 m 3 s |
| 530 000 | 1 767.9 | 1 h 6 m 56.2 s |
| 540 000 | 1 760.1 | 1 h 7 m 49.6 s |
| 550 000 | 1 752.4 | 1 h 8 m 43.3 s |
| 560 000 | 1 744.8 | 1 h 9 m 37.2 s |
| 570 000 | 1 737.4 | 1 h 10 m 31.3 s |
| 580 000 | 1 730.0 | 1 h 11 m 25.7 s |
| 590 000 | 1 722.7 | 1 h 12 m 20.2 s |
| 600 000 | 1 715.5 | 1 h 13 m 15.1 s |
| 610 000 | 1 708.4 | 1 h 14 m 10.1 s |
| 620 000 | 1 701.4 | 1 h 15 m 5.4 s |
| 630 000 | 1 694.5 | 1 h 16 m 0.9 s |
| 640 000 | 1 687.6 | 1 h 16 m 56.6 s |
| 650 000 | 1 680.9 | 1 h 17 m 52.6 s |
| 660 000 | 1 674.2 | 1 h 18 m 48.8 s |
| 670 000 | 1 667.6 | 1 h 19 m 45.2 s |
| 680 000 | 1 661.0 | 1 h 20 m 41.8 s |
| 690 000 | 1 654.6 | 1 h 21 m 38.7 s |
| 700 000 | 1 648.2 | 1 h 22 m 35.7 s |
| 710 000 | 1 641.9 | 1 h 23 m 33 s |
| 720 000 | 1 635.7 | 1 h 24 m 30.5 s |
| 730 000 | 1 629.5 | 1 h 25 m 28.3 s |
| 740 000 | 1 623.4 | 1 h 26 m 26.2 s |
| 750 000 | 1 617.4 | 1 h 27 m 24.4 s |
| 760 000 | 1 611.4 | 1 h 28 m 22.8 s |
| 770 000 | 1 605.6 | 1 h 29 m 21.4 s |
| 780 000 | 1 599.7 | 1 h 30 m 20.2 s |
| 790 000 | 1 594.0 | 1 h 31 m 19.2 s |
| 800 000 | 1 588.3 | 1 h 32 m 18.4 s |
| 810 000 | 1 582.6 | 1 h 33 m 17.9 s |
| 820 000 | 1 577.0 | 1 h 34 m 17.5 s |
| 830 000 | 1 571.5 | 1 h 35 m 17.4 s |
| 840 000 | 1 566.0 | 1 h 36 m 17.5 s |
| 850 000 | 1 560.6 | 1 h 37 m 17.8 s |
| 860 000 | 1 555.3 | 1 h 38 m 18.3 s |
| 870 000 | 1 550.0 | 1 h 39 m 19 s |
| 880 000 | 1 544.7 | 1 h 40 m 19.9 s |
| 890 000 | 1 539.5 | 1 h 41 m 21 s |
| 900 000 | 1 534.4 | 1 h 42 m 22.3 s |
| 910 000 | 1 529.3 | 1 h 43 m 23.8 s |
| 920 000 | 1 524.3 | 1 h 44 m 25.6 s |
| 930 000 | 1 519.3 | 1 h 45 m 27.5 s |
| 940 000 | 1 514.3 | 1 h 46 m 29.6 s |
| 950 000 | 1 509.5 | 1 h 47 m 32 s |
| 960 000 | 1 504.6 | 1 h 48 m 34.5 s |
| 970 000 | 1 499.8 | 1 h 49 m 37.2 s |
| 980 000 | 1 495.1 | 1 h 50 m 40.2 s |
| 990 000 | 1 490.3 | 1 h 51 m 43.3 s |
| 1 000 000 | 1 485.7 | 1 h 52 m 46.7 s |
| 2 868 378 | 1 009.1 | 5 h 59 m 56.5 s |
| 8 140 000 | 635.7 | 3 d 5 h 59 m 49.6 s |