Difference between revisions of "Cheat sheet/nl"

Revision as of 20:13, 15 July 2013 (view source) Guest91111youtube (talk | contribs) (Created page with "(Deze pagina wordt nog vertaald) Kerbal space program raketwetenschappers '''cheat sheet''': Delta-v kaarten, formules en meer voor jou referentie, zodat je van hier naar daar...")		Revision as of 19:44, 8 August 2013 (view source) XZise (talk | contribs) m (-math tags;) Newer edit →
Line 7:		Line 7:
	Dit is de tweede wet van Newtion. Als de ratio kleiner dan 1 is, zal het voertuig niet van de grond komen. Je moet dan wel rekening houden met de zwaartekracht van de lanceerplaats.		Dit is de tweede wet van Newtion. Als de ratio kleiner dan 1 is, zal het voertuig niet van de grond komen. Je moet dan wel rekening houden met de zwaartekracht van de lanceerplaats.
−	{{Formula|math=<math>\text{TWR} = \frac{F_T}{m \cdot g} > 1</math>|where=* <math>F_T</math> Kracht van de motoren	+	{{Formula|math=\text{TWR} = \frac{F_T}{m \cdot g} > 1|where=* <math>F_T</math> Kracht van de motoren
	* <math>m</math> Totale massa van het object		* <math>m</math> Totale massa van het object
	* <math>g</math> Lokale versnelling van de zwaartekracht (Oppervlaktezwaartekracht)}}		* <math>g</math> Lokale versnelling van de zwaartekracht (Oppervlaktezwaartekracht)}}
Line 21:		Line 21:
	{{See also|Tutorial:Advanced Rocket Design}}		{{See also|Tutorial:Advanced Rocket Design}}
	Basic calculation of a rocket's &Delta;v. Use the atmospheric and vacuum thrust values for atmospheric and vacuum &Delta;v, respectively.		Basic calculation of a rocket's &Delta;v. Use the atmospheric and vacuum thrust values for atmospheric and vacuum &Delta;v, respectively.
−	{{Formula|math=<math>\Delta{v} = ln\left(\frac{M_{start}}{M_{end}}\right) \cdot I_{sp} \cdot 9.81 \frac{m}{s^2}</math>|where=* <math>\Delta{v}</math> Is de verandering van snelheid in m/s	+	{{Formula|math=\Delta{v} = ln\left(\frac{M_{start}}{M_{end}}\right) \cdot I_{sp} \cdot 9.81 \frac{m}{s^2}|where=* <math>\Delta{v}</math> Is de verandering van snelheid in m/s
	Basis berekening voor een raket is de &Delta;v. Gebruik de Atmosferische waarden voor in de Atmosfeer, en de vacuum waarden voor in de ruimte.		Basis berekening voor een raket is de &Delta;v. Gebruik de Atmosferische waarden voor in de Atmosfeer, en de vacuum waarden voor in de ruimte.
	* <math>M_{start}</math> Is de start massa van de raket <math>M_{end}</math>		* <math>M_{start}</math> Is de start massa van de raket <math>M_{end}</math>

---

Revision as of 19:44, 8 August 2013

(Deze pagina wordt nog vertaald) Kerbal space program raketwetenschappers cheat sheet: Delta-v kaarten, formules en meer voor jou referentie, zodat je van hier naar daar en weer terug kan

Wiskunde

Kracht/Gewicht Ratio (TWR)

→ See also: Thrust-to-weight ratio

Dit is de tweede wet van Newtion. Als de ratio kleiner dan 1 is, zal het voertuig niet van de grond komen. Je moet dan wel rekening houden met de zwaartekracht van de lanceerplaats.

Gecombineerde specifieke stoot (I_sp)

→ See also: Specific impulse

Als de I<subsp</sub> hetzelfde is voor alle motoren in een stage, dan is de I_sp gelijk aan die van een motor van hetzelfde soort. Als de I_sp verschillend is voor de motoren in een stage, gebruik je de volgende formule:

${\displaystyle I_{sp}={\frac {(F_{1}+F_{2}+\dots )}{{\frac {F_{1}}{I_{sp1}}}+{\frac {F_{2}}{I_{sp2}}}+\dots }}}$

Delta-v (Δv)

Basis berekening

→ See also: Tutorial:Advanced Rocket Design

Basic calculation of a rocket's Δv. Use the atmospheric and vacuum thrust values for atmospheric and vacuum Δv, respectively.

{M_{end}}\right) \cdot I_{sp} \cdot 9.81 \frac{m}{s^2}|where=* ${\displaystyle \Delta {v}}$ Is de verandering van snelheid in m/s

Basis berekening voor een raket is de Δv. Gebruik de Atmosferische waarden voor in de Atmosfeer, en de vacuum waarden voor in de ruimte.

• ${\displaystyle M_{start}}$ Is de start massa van de raket ${\displaystyle M_{end}}$
• ${\displaystyle M_{end}}$ Is de massa van de raket zonder brandstof ${\displaystyle M_{start}}$
• ${\displaystyle I_{sp}}$ Is de specifieke stoot van de motoren in seconden.}}

Echte Δv van een stage die begint in de atmosfeer op het lanceerplatform en eindigt in de ruimte

Body	Δvout
Kerbin	1000 m/s2
Geen verdere data bekend

Berekenen van de Δv van een raket stage kan een ander getal geven als de raket nog moet beginnen in de atmosfeer, bijvoorbeeld op het lanceerplatform. Δv_out is the hoeveelheid Δv die nodig is om de atmosfeer van een hemellichaam te verlaten, NIET om orbit te bereikwn. Deze formule is handig om uit te vinden of de hoeveelheid Δv in de Transition stage genoeg is om van de atmosfeer in de ruimte te komen.

${\displaystyle \Delta {v}_{T}={\frac {\Delta {v}_{atm}-\Delta {v}_{out}}{\Delta {v}_{atm}}}\cdot \Delta {v}_{vac}+\Delta {v}_{out}}$

Maps

Een fan made map die laat zien hoeveel Δv nodig is om naar een andere plek te gaan.

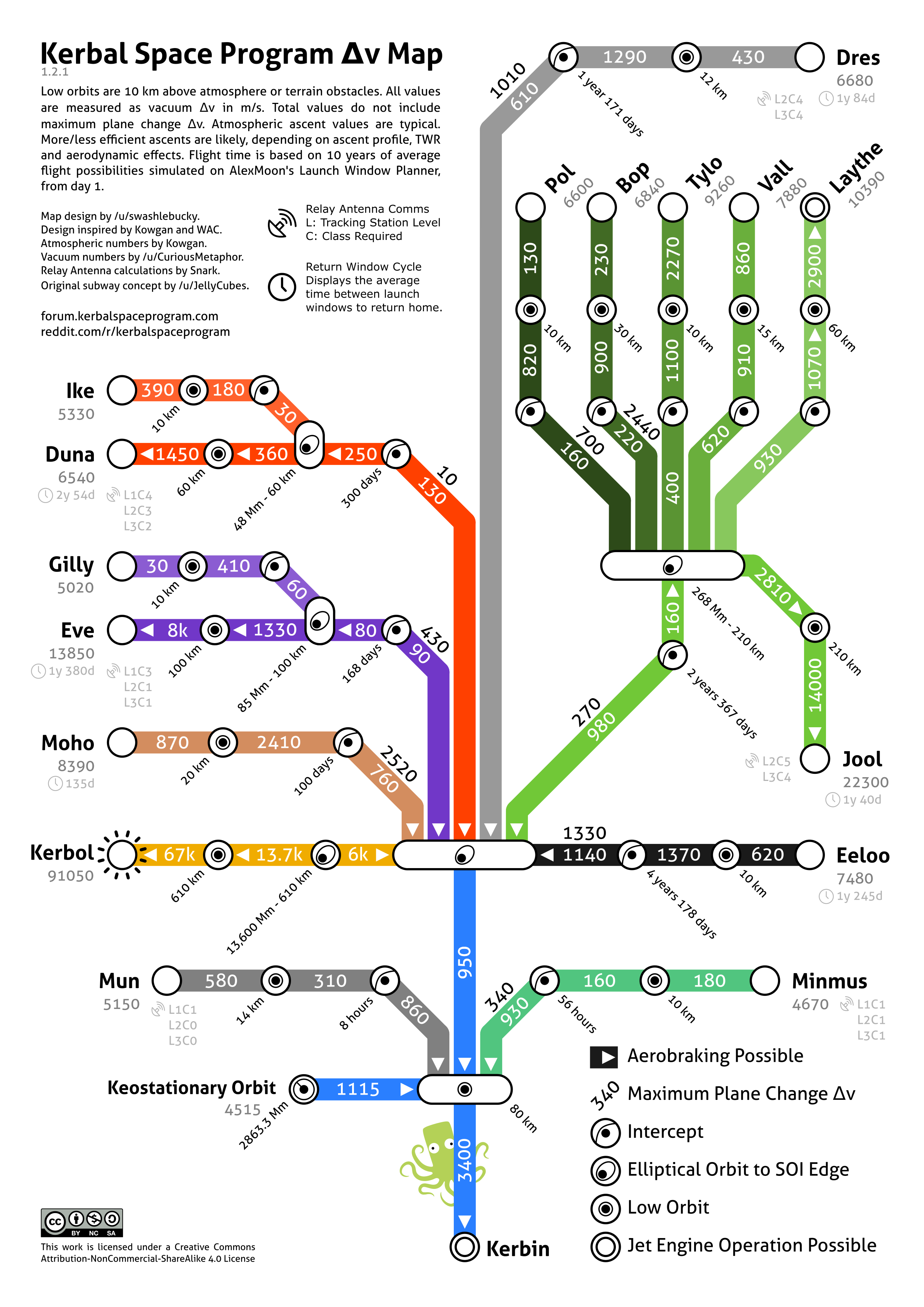
Total Δv values

• http://www.skyrender.net/lp/ksp/system_map.png

Δv change values

• http://i.imgur.com/duY2S.png

Δv nomogram

• http://ubuntuone.com/1kD39BCoV38WP1QeG6MtO6

Math examples

TWR

1. This is Newton's Second Law.
2. If the ratio is less than 1 the craft will not lift off the ground.

• Equation:

${\displaystyle {\text{TWR}}={\frac {F}{m\cdot g}}}$

• Simplified:

TWR = F / (m * g) > 1

• Explained:

TWR = Force of Thrust / ( Total Mass X 9.81 ) > 1

• Example:

200 kilonewton rocket engine on a 15 ton rocket launching from Kerbin Space Center.

TWR = 200 kN / ( 15 Tons total Mass X 9.81 m/s2 ) = 1.36 which is > 1 which means liftoff!

I_sp

1. When I_sp is the same for all engines in a stage, then the I_sp is equal to a single engine. So six 200 I_sp engines still yields only 200 I_sp.
2. When I_sp is different for engines in a single stage, then use the following equation:

• Equation:

${\displaystyle I_{sp}={\frac {(F_{1}+F_{2}+\dots )}{{\frac {F_{1}}{I_{sp1}}}+{\frac {F_{2}}{I_{sp2}}}+\dots }}}$

• Simplified:

I_sp = ( F1 + F2 + ... ) / ( ( F1 / I_sp1 ) + ( F2 / I_sp2 ) + ... )

• Explained:

I_sp = ( Force of Thrust of 1st Engine + Force of Thrust of 2nd Engine...and so on... ) / ( ( Force of Thrust of 1st Engine / I_sp of 1st Engine ) + ( Force of Thrust of 2nd Engine / I_sp of 2nd Engine ) + ...and so on... )

• Example:

Two engines, one rated 200 newtons and 120 seconds I_sp ; another engine rated 50 newtons and 200 seconds I_sp.

Isp = (200 newtons + 50 newtons) / ( ( 200 newtons / 120 ) + ( 50 newtons / 200 ) = 130.89 seconds I_sp

Δv

1. For atmospheric Δv value, use atmospheric thrust values.
2. For vacuum Δv value, use vacuum thrust values.
3. Use this equation to figure out the Δv per stage:

• Equation:

${\displaystyle \Delta {v}=ln\left({\frac {M_{start}}{M_{dry}}}\right)\cdot I_{sp}\cdot 9.81{\frac {m}{s^{2}}}}$

• Simplified:

Δv = ln ( Mstart / Mdry ) * I_sp * g

• Explained:

Δv = ln ( Starting Mass / Dry Mass ) X Isp X 9.81

• Example:

Single Stage Rocket that weighs 23 tons when full, 15 tons when fuel is emptied, and engine that outputs 120 seconds I_sp.

Δv = ln ( 23 Tons / 15 Tons ) × 120 seconds I_sp × 9.81m/s = Total Δv of 1803.2 m/s2

True Δv

1. How to calculate the Δv of a rocket stage that transitions from Kerbin atmosphere to vacuum.
2. Assumption: It takes approximately 1000 m/s2 of Δv to escape Kerbin's atmosphere before vacuum Δv values take over for the stage powering the transition.
3. Note: This equation is an guess, approximation, and is not 100% accurate. Per forum user stupid_chris who came up with the equation: "The results will vary a bit depending on your TWR and such, but it should usually be pretty darn accurate."

• Equation for Kerbin Atmospheric Escape:

${\displaystyle \Delta {v}_{T}={\frac {\Delta {v}_{atm}-\Delta {v}_{out}{\frac {m}{s^{2}}}}{\Delta {v}_{atm}}}\cdot \Delta {v}_{vac}+\Delta {v}_{out}{\frac {m}{s^{2}}}}$

• Simplified:

True Δv = ( ( Δv atm - 1000 ) / Δv atm ) * Δv vac + 1000

• Explained:

True Δv = ( ( Total Δv in atmosphere - 1000 m/s2) / Total Δv in atmosphere ) X Total Δv in vacuum + 1000

• Example:

Single Stage with total atmospheric Δv of 5000 m/s2, and rated 6000 Δv in vacuum.

Transitional Δv = ( ( 5000 Δv atm - 1000 Δv Required to escape Kerbin atmosphere ) / 5000 Δv atm ) X 6000 Δv vac + 1000 Δv Required to escape Kerbin atmosphere = Total Δv of 5800 m/s2

See also

• Tutorials
• Terminology
• The Drawing Board: A library of tutorials and other useful information