Difference between revisions of "Atmosphere"
m (→Terminal velocity: *align some formula;) |
Grande1900 (talk | contribs) m |
||
| (26 intermediate revisions by 16 users not shown) | |||
| Line 1: | Line 1: | ||
| + | [[File:Pressures.svg|thumb|The pressures for all atmospheres]] | ||
{| class="wikitable float-right" | {| class="wikitable float-right" | ||
| − | ! colspan="2" | | + | ! colspan="4" | Planets |
| + | ! style="border-left-width:2px;" colspan="2" | Moons | ||
|- | |- | ||
| style="border-right:0px;" | [[File:TinyEve.png|16px]] | | style="border-right:0px;" | [[File:TinyEve.png|16px]] | ||
| style="border-left:0px;" | [[Eve]] | | style="border-left:0px;" | [[Eve]] | ||
| − | |||
| style="border-right:0px;" | [[File:TinyKerbin.png|16px]] | | style="border-right:0px;" | [[File:TinyKerbin.png|16px]] | ||
| style="border-left:0px;" | [[Kerbin]] | | style="border-left:0px;" | [[Kerbin]] | ||
| + | | style="border-left-width:2px; border-right:0px; vertical-align:top;" rowspan="2" | [[File:TinyLaythe.png|16px]] | ||
| + | | style="border-left:0px; vertical-align:top;" rowspan="2" | [[Laythe]] | ||
|- | |- | ||
| style="border-right:0px;" | [[File:TinyDuna.png|16px]] | | style="border-right:0px;" | [[File:TinyDuna.png|16px]] | ||
| style="border-left:0px;" | [[Duna]] | | style="border-left:0px;" | [[Duna]] | ||
| − | |||
| style="border-right:0px;" | [[File:TinyJool.png|16px]] | | style="border-right:0px;" | [[File:TinyJool.png|16px]] | ||
| style="border-left:0px;" | [[Jool]] | | style="border-left:0px;" | [[Jool]] | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|} | |} | ||
| − | The '''atmosphere''' of a celestial body slows the movement of any object passing through it, a force known as atmospheric drag (or simply '''drag'''). An atmosphere also allows for aerodynamic lift. The celestial bodies with atmospheres are the planets [[Eve]], [[Kerbin]], [[Duna]] and [[Jool]], as well as [[Laythe]], a moon of Jool. Only [[Kerbin]] and [[Laythe]] have atmospheres that contain oxygen. | + | The '''atmosphere''' of a celestial body slows the movement of any object passing through it, a force known as atmospheric drag (or simply '''drag'''). An atmosphere also allows for aerodynamic lift. The celestial bodies with atmospheres are the planets [[Eve]], [[Kerbin]], [[Duna]] and [[Jool]], as well as [[Laythe]], a moon of Jool. Only [[Kerbin]] and [[Laythe]] have atmospheres that contain oxygen and thus produce [[intake air]] for [[jet engine]]s to work, as well as allowing Kerbals to take off their helmets. |
| − | + | Atmospheres allow [[aerobraking]] and easier landing. However, an atmosphere makes taking off from a planet more difficult and increases the minimum stable orbit altitude. | |
| − | Atmospheres | + | == Physical quantities == |
| + | Atmospheres have a '''pressure''' that varies in an exponential way with the increasing altitude: | ||
| + | : <math>p = p_0 \cdot e^\frac{-altitude}{H}</math> | ||
| − | + | where ''p<sub>0</sub>'' is the atmosphere's pressure (Pa) at altitude 0, and ''H'' is the scale height (m). | |
| − | + | The ''scale height'' of an atmosphere define at which rate the pressure drops with altitude. In the case of Kerbin's atmosphere, the scale height is about 5600m and means that the atmospheric pressure will be 2.718 times smaller each time you go 5600m higher. | |
| − | |||
| − | + | Atmospheres also vary in '''temperature'''. Though this has little effect on gameplay, it affects the atmospheric density and the speed of sound both used in drag calculations. With the [[w:Ideal_gas_law|Ideal gas law]] the density (''ρ'') in kg/m<sup>3</sup> is calculated with the equation: | |
| − | : <math> | + | <!-- Bypassing the fact that parsing math equations dont work. Replace with the math attributes when they will work.--> |
| + | [[File:DensityEq.png]] | ||
| + | <!--: <math>\rho = \frac{p}{R \cdot T}</math>--> | ||
| − | where '' | + | where ''R'' is the [[w:Gas_constant#Specific_gas_constant|specific gas constant]] equal to 287.053 J/kg-K. |
| − | + | The speed of sound (c) is calculated with: | |
| − | : <math> | + | <!-- Bypassing the fact that parsing math equations dont work. Replace with the math attributes when they will work.--> |
| + | [[File:SpeedOfSoundEq.png]] | ||
| + | <!--: <math>c = \sqrt{1.4 \cdot R \cdot T}</math>--> | ||
| − | + | Note that for Kerbin atmosphere, the pressure and temperature are modeled to match Earth atmosphere by using [[w:U.S._Standard_Atmosphere|U.S. Standard Atmosphere]] (USSA) equations (see [[Kerbin#Atmosphere|Kerbin atmosphere]]). | |
| − | |||
| − | : | + | == Drag == |
| + | [[File:Mk16-XL Parachute.jpg|thumb|right|A Mk1-2 pod with a Mk16-XL parachute being slowed by drag in Kerbin's atmosphere.]] | ||
| + | In the game, the force of atmospheric '''drag''' is dependent on the shape of a part and which of the part's attachment nodes are in use. For example, nose cones reduce drag on the part to which they are attached. A debug-mode option allows you to see the exact drag force on a given part by right-clicking on it. | ||
| − | : <math>\rho | + | Generally, the drag works with the following equation: |
| + | : <math>F_D = 0.5\, \rho\, v^2\, d\, A</math> | ||
| − | where | + | where ''ρ'' is the atmospheric density (kg/m<sup>3</sup>), ''v'' is the ship's velocity (m/s), ''d'' is the coefficient of drag (dimensionless), and ''A'' is the [[w:cross section (geometry)|cross-sectional area]] (m<sup>2</sup>). |
| − | |||
| − | + | == Atmospheric height == | |
| + | The '''atmospheric height''' depends on the scale height of the celestial body and is where 0.000001<sup>th</sup> (0.0001%) of the surface pressure remains. Therefore, the atmospheric pressure at the edge of the atmosphere is relative; for example a craft in orbit around Jool can have a lower orbit (relative to the surface) because the surface pressure is higher. | ||
| + | :<math>alt_{\text{atmospheric height}} = -ln\left(10^{-6}\right) \cdot \text{scale height}</math> | ||
| + | :<math>p_{\text{atmospheric height}} = p_0 \cdot 10^{-6}</math> | ||
| + | To calculate the atmospheric heights of other celestial bodies: | ||
| + | :<math>alt_{\text{atmospheric height (real)}} = -ln\left(\frac{10^{-6}}{p_0}\right) \cdot \text{scale height}</math> | ||
| − | |||
== Terminal velocity == | == Terminal velocity == | ||
| − | + | {{See also||{{Wikipedia|terminal velocity}}}} | |
| + | The '''terminal velocity''' of an object falling through an atmosphere is the velocity at which the force of gravity is equal to the force of drag. Terminal velocity changes as a function of altitude. Given enough time, an object falling into the atmosphere will slow to terminal velocity and then remain at terminal velocity for the rest of its fall. | ||
Terminal velocity is important because: | Terminal velocity is important because: | ||
| Line 70: | Line 78: | ||
where ''m'' is still the ship's mass, ''G'' is the [[Template:G|gravitational constant]], ''M'' is the mass of the planet, and ''r'' is the distance from the ''center'' of the planet to the falling object. | where ''m'' is still the ship's mass, ''G'' is the [[Template:G|gravitational constant]], ''M'' is the mass of the planet, and ''r'' is the distance from the ''center'' of the planet to the falling object. | ||
| − | + | The terminal velocity can be found by finding for what speed ''F<sub>G</sub>'' is equal to ''F<sub>D</sub>''. | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
=== Examples === | === Examples === | ||
| + | The terminal velocity for an given imaginary body depends from the altitude on the different celestial bodies. | ||
{| class="wikitable" | {| class="wikitable" | ||
!rowspan=2 valign=bottom| Altitude (m) ||colspan=6| v<sub>T</sub> (m/s) | !rowspan=2 valign=bottom| Altitude (m) ||colspan=6| v<sub>T</sub> (m/s) | ||
| Line 106: | Line 97: | ||
== On-rails physics == | == On-rails physics == | ||
| − | + | A ship is "'''on rails'''" when it's no longer the primary focus of the simulation, which occurs when it's further than 2.25 km from the actively-controlled ship. If such a ship have its orbit passing through a planet's atmosphere, one of two things will happen based on atmospheric pressure at the ship's altitude: | |
* below 0.01 atm: no atmospheric drag will occur — the ship will be completely unaffected | * below 0.01 atm: no atmospheric drag will occur — the ship will be completely unaffected | ||
| Line 116: | Line 107: | ||
! Body || Altitude (m) | ! Body || Altitude (m) | ||
|- | |- | ||
| − | | [[Eve]] ||align="right"| {{Formatnum|{{ | + | | [[Eve]] ||align="right"| {{Formatnum|{{Body data/alt at pressure|pressure=0.01|body=Eve}}}} |
|- | |- | ||
| − | | [[Kerbin]] ||align="right"| {{Formatnum|{{ | + | | [[Kerbin]] ||align="right"| {{Formatnum|{{Body data/alt at pressure|pressure=0.01|body=Kerbin}}}} |
|- | |- | ||
| − | | [[Duna]] ||align="right"| {{Formatnum|{{ | + | | [[Duna]] ||align="right"| {{Formatnum|{{Body data/alt at pressure|pressure=0.01|body=Duna}}}} |
|- | |- | ||
| − | | [[Jool]] ||align="right"| {{Formatnum|{{ | + | | [[Jool]] ||align="right"| {{Formatnum|{{Body data/alt at pressure|pressure=0.01|body=Jool}}}} |
|- | |- | ||
| − | | [[Laythe]] ||align="right"| {{Formatnum|{{ | + | | [[Laythe]] ||align="right"| {{Formatnum|{{Body data/alt at pressure|pressure=0.01|body=Laythe}}}} |
|} | |} | ||
| − | == | + | == See also == |
| − | + | * [[Atmospheric entry]] | |
| − | |||
| − | |||
| − | |||
| − | |||
== Notes == | == Notes == | ||
<references /> | <references /> | ||
| + | |||
| + | [[Category:Celestials]] | ||
Latest revision as of 02:09, 4 November 2022
| Planets | Moons | ||||
|---|---|---|---|---|---|
| |
Eve | |
Kerbin | |
Laythe |
| |
Duna | |
Jool | ||
The atmosphere of a celestial body slows the movement of any object passing through it, a force known as atmospheric drag (or simply drag). An atmosphere also allows for aerodynamic lift. The celestial bodies with atmospheres are the planets Eve, Kerbin, Duna and Jool, as well as Laythe, a moon of Jool. Only Kerbin and Laythe have atmospheres that contain oxygen and thus produce intake air for jet engines to work, as well as allowing Kerbals to take off their helmets.
Atmospheres allow aerobraking and easier landing. However, an atmosphere makes taking off from a planet more difficult and increases the minimum stable orbit altitude.
Contents
Physical quantities
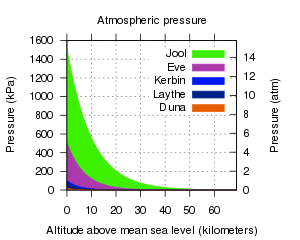
Atmospheres have a pressure that varies in an exponential way with the increasing altitude:
where p0 is the atmosphere's pressure (Pa) at altitude 0, and H is the scale height (m).
The scale height of an atmosphere define at which rate the pressure drops with altitude. In the case of Kerbin's atmosphere, the scale height is about 5600m and means that the atmospheric pressure will be 2.718 times smaller each time you go 5600m higher.
Atmospheres also vary in temperature. Though this has little effect on gameplay, it affects the atmospheric density and the speed of sound both used in drag calculations. With the Ideal gas law the density (ρ) in kg/m3 is calculated with the equation:
where R is the specific gas constant equal to 287.053 J/kg-K.
The speed of sound (c) is calculated with:
Note that for Kerbin atmosphere, the pressure and temperature are modeled to match Earth atmosphere by using U.S. Standard Atmosphere (USSA) equations (see Kerbin atmosphere).
Drag
In the game, the force of atmospheric drag is dependent on the shape of a part and which of the part's attachment nodes are in use. For example, nose cones reduce drag on the part to which they are attached. A debug-mode option allows you to see the exact drag force on a given part by right-clicking on it.
Generally, the drag works with the following equation:
where ρ is the atmospheric density (kg/m3), v is the ship's velocity (m/s), d is the coefficient of drag (dimensionless), and A is the cross-sectional area (m2).
Atmospheric height
The atmospheric height depends on the scale height of the celestial body and is where 0.000001th (0.0001%) of the surface pressure remains. Therefore, the atmospheric pressure at the edge of the atmosphere is relative; for example a craft in orbit around Jool can have a lower orbit (relative to the surface) because the surface pressure is higher.
To calculate the atmospheric heights of other celestial bodies:
Terminal velocity
- → See also: terminal velocity on Wikipedia
The terminal velocity of an object falling through an atmosphere is the velocity at which the force of gravity is equal to the force of drag. Terminal velocity changes as a function of altitude. Given enough time, an object falling into the atmosphere will slow to terminal velocity and then remain at terminal velocity for the rest of its fall.
Terminal velocity is important because:
- It describes the amount of velocity which a spacecraft must burn away when it is close to the ground.
- It represents the speed at which a ship should be traveling upward during a fuel-optimal ascent.
The force of gravity (FG) is:
where m is still the ship's mass, G is the gravitational constant, M is the mass of the planet, and r is the distance from the center of the planet to the falling object.
The terminal velocity can be found by finding for what speed FG is equal to FD.
Examples
The terminal velocity for an given imaginary body depends from the altitude on the different celestial bodies.
| Altitude (m) | vT (m/s) | |||||
|---|---|---|---|---|---|---|
| Eve | Kerbin | Duna | Jool | Laythe | ||
| 0 | 58.385 | 100.13 | 212.41 | 23.124 | 115.62 | |
| 100 | 58.783 | 101.01 | 214.21 | 23.162 | 116.32 | |
| 1000 | 62.494 | 109.30 | 231.16 | 23.508 | 122.83 | |
| 10000 | 115.27 | 240.52 | 495.18 | 27.272 | 211.77 | |
On-rails physics
A ship is "on rails" when it's no longer the primary focus of the simulation, which occurs when it's further than 2.25 km from the actively-controlled ship. If such a ship have its orbit passing through a planet's atmosphere, one of two things will happen based on atmospheric pressure at the ship's altitude:
- below 0.01 atm: no atmospheric drag will occur — the ship will be completely unaffected
- 0.01 atm or above: the ship will disappear
The following table gives the altitude of this 0.01 atm threshold for each celestial body with an atmosphere:
| Body | Altitude (m) |
|---|---|
| Eve | 44 745 |
| Kerbin | 25 789 |
| Duna | 10 814 |
| Jool | 219 397 |
| Laythe | 32 755 |